THE VISCOUS FORCE

John W. Dooley, Physics Department, Millersville University

A force, F, pulls a plate a velocity across a viscous

fluid. The lower plate is fixed.<

To a large extent, the force is independent of the material used for the moving plates. However, it does depend on the nature of the viscous fluid, and fluids are characterized individually by their viscous effect.

The force by the fluid on the upper plate is directed to the left.

We will express the relation between force and velocity in terms of the force per unit area on the upper plate by the fluid. The experimental result is

The constant of proportionality, , is called the viscosity. is different for each different fluid. It also depends on fluid temperature, typically becoming smaller at higher temperature for liquids, but larger at high temperature for gases.

A table of some typical viscosites at room temperature.

The units of viscosity are

In practice the unit used is the poise (named for the French physicist J.L. Poiseuille):

(The poise is the natural viscosity unit in the cgs system of units.)

STEADY STATE VELOCITY FIELD FOR A VISCOUS FLUID BETWEEN MOVING PLATES

When the experiment described above is first begun, the velocity field is time dependent. If the field evolves to one in which the net force on a unit volume is zero, this field will not change. This condition is called the steady state condition. (It is not equilibrium, because the fluid is in motion.)

We approach this by considering a variation of the experiment above. First we imagine changing frames of reference, to one which at rest on plate A, rather than plate B. From there, we see the plate B moving left at a constant velocity .

Using the rule for viscous force, we conclude that the force on plate B by the fluid is the same magnitude as the fluid force on plate A, but directed to the right. (For relative motion with constant velocity, observers agree concerning the accelerations of and forces on objects.)

The viscous fluid exerts a net force of zero on plate B.

In order to have done that experiment, we had to exert a force to the left on plate B, to "hold it in place." We can imagine another experiment, in which a viscous force holds plate B in place. We build it as shown in the figure. Plate C moves to the left and the fluid below exerts a force to the left on plate B. Since the distances between plates are the same, and both velocities have the same magnitude, the net force on plate B is zero.

The viscous fluid exerts a net force of zero on plate B,

as viewed in a frame of reference with C stationary.

Now we shift coordinate systems again, to one at rest on plate C. Now the motion looks to us as shown in the figure. The forces are unchanged, so the net force on plate B remains zero.

We replace plate B with a slab of fluid. Since the forces acting across the surface are independent of the content of the surface, the net force on our slab of fluid remains zero. The velocity of the fluid remains constant. We have found the steady state velocity half way between plates A and C.

Velocity field for a viscous fluid between two plates, one

of which is stationary.

We can repeat this thought experiment. At each stage, we can show that half way between a moving slab of fluid and the bottom plate (at rest), the velocity is half that of the upper slab. This just says that the velocity field is proportional to the height above the bottom slab. The constant of proportionality is chosen so that the fluid has the correct velocity on at the top plate. (There, as on the bottom, the fluid velocity equals the plate velocity.) We can write

.

MECHANICAL ENERGY IS NOT CONSERVED IN VISCOUS FLOW

The kinetic energy of every bit of fluid is constant in the steady state situation pictured above. Yet work is being done by the plate at a rate

LAMINAR FLOW

Within the fluid, we may visualize surfaces of constant velocity. In this case they are planes parallel to the plates, as shown above. We may think of layers of fluid, defined by the condition that each bit of fluid in the layer has the same velocity. Each layer contains a family of streamlines.

The rate at which work is done by the bottom surface of a layer is . is the force by the bottom on the fluid below, and is the velocity of the layer.) The rate at which work is done on the next layer below is where is the velocity of the layer below.

In the figure above, , and we conclude that energy is dissipated in the interaction between the two layers.

LOCAL EXPRESSION FOR THE VISCOUS FORCE

The force by a viscous fluid on the moving upper plate.

The viscous force by the fluid on a unit area of the upper plate was represented as

.

To calculate this force we need to know the separation of the two plates, D. This makes it appear that a distant object is the direct source of the force on the top plate, when in fact it is the fluid just below the plate which exerts the force. Now that we know that the velocity is simply proportional to the height above the lower plate, we know that

.

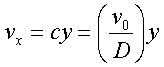
Thus

and we can write

.

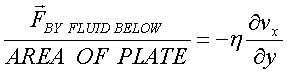
This is an expression that we can apply even if we do not know the distance D, as long as we know the local spatial derivative of the velocity. We can use it for a barge being pulled across a lake whose depth we do not know. We have a local expression for the viscous force.

VISCOUS FORCE ON A UNIT VOLUME IN LAMINAR FLOW

The force on a volume of fluid by neighboring fluid above and

below is determined by the velocity derivatives above and below

We choose our sample volume so that its shape conforms to the shape of the layers in laminar flow. An example for plane laminar flow is shown in the figure. The upper surface moves slightly faster than the lower. We imagine that the volume moves at the velocity of its center.

In the figure, the force by the fluid above on our volume is

.

where is the area of the top of our volume. Note the positive sign, because the faster upper fluid is pulling our volume towards the right.

The net viscous force is

,

where is the area of the top (and bottom) of our volume.

Note that the fluid on the bottom moves slower, and pulls to the left. If the velocity derivative is the same, top and bottom, the net force is zero. This is the steady state situation if the bottom plate is fixed and the top plate moves at constant velocity, making the derivative of the velocity constant.

To find the force per unit volume we assign a height to the volume in the picture. Then the volume of our sample is , and we can write the force per unit volume:

.

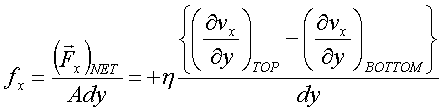
In the limit as , this becomes

.

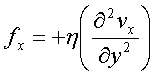
If the density is constant, this result can be extended to three dimensions:

.

Note that is a scalar operator. The vector character of the expression is carried by the velocity vector.

Euler's equation for an incompressible viscous fluid becomes

When the viscosity is included, this is known as the Navier-Stokes equation for an incompressible fluid.

Return to index.

Return to survey.

Go forward to Poiseuille's Law.