
Go directly to derivation of several forms of strain.
The same forces that hold a drop of water together in a weightless environment are at play in solids. If the sample is compressed, both fluids and solids resist compression with similar strength, although solids are generally less compressible. Solids are much more resistant to stretching than liquids. (If the idea of stretching a liquid is new, consider the forces at play at the highest part of a siphon tube.)
The idea of pressure remains useful in studying solids. It is renamed "stress" and (unlike pressure for fluids) its sign is now taken to be negative if the stress tends to reduce the volume of a sample. It still has units of Newtons per square meter, or Pascals.
Here, we will emphasize a different aspect of the response of a system to applied pressure. In the case of fluids we had considered the flow which results from pressure gradients, and ignored the change in density that pressure causes. Now, for solids (and incidentally for fluids) we will focus upon the change in volume (hence density) change that pressure causes in a sample. For the time being, we will ignore accelerations caused by pressure.
It is found experimentally that when a uniform pressure (the same on all faces) is applied to a sample, the sample volume changes. If we continue to apply the stress, the volume does not continue to change; it remains at a constant value. If the stress is removed, the sample returns to its original volume.
Experimentally, the fractional change in volume of a sample is proportional to the pressure. This response is analogous to the response of a spring to an applied force. (Just as for a spring, the proportionality fails at very large stress, but is quite useful in ordinary situations.) The fractional change in volume is called a strain.
The proportionality can be represented in two ways:
in which
is called the bulk modulus, or
in which
is called the compressibility.
Recall that positive stress tends to increase the volume of the sample.
For typical materials
is measured in units of
,
and
is measured
in units of
.
Each material has its own value for
,
and for ordinary materials
ranges from
to
.
Since solids maintain their shape without need of a container, it is easy to apply a non-uniform stress to a solid. For example, a pair of forces can be applied to the left and right ends of a sample. The two forces are equal in magnitude and opposite in direction, so that the sample does not accelerate. If both forces pull, the applied stress is said to be a tensile stress.
For the uniaxial stress, it would be incorrect to calculate the change in volume using the bulk modulus. This is because under this stress, the sides of the sample tend to shrink in. (An extreme example of this "side effect" may be seen by squeezing a balloon between two boards. The water swells out to the side, increasing the sideways dimension of the balloon.)
Of more practical interest for a uniaxial tensile stress is the change in length of the sample. The fractional change in length is found to be proportional to the applied stress:
Where we use the symbol
for a uniaxial stress, reserving
for uniform pressure. The units of
are still Newton/square meter, or Pa. The constant of proportionality,
, is called Young's
modulus. The fractional change in length is called the tensile
strain.
For anisotropic materials, such as wood, the value of Young's modulus depends on the direction of the applied stress. (It is plausible that wood pulled parallel to the grain will stretch differently from wood pulled perpendicular to the grain.) For now we will consider isotropic materials, such as plastic, in which the direction of the stress is not significant.
The side effect is
also of interest. It is found that the side effect change in width of the
sample is proportional to the direct effect change in length, and has the
opposite sense. The ratio of the fractional change in width to the fractional
change in length is called Poisson's ratio, :
If the sample is incompressible, so that its change in volume must be
zero, then .
More compressible materials have smaller values for Poisson's ratio.
It turns out that the three elastic constants,
are related. For an isotropic material the relation is:
Typically the bulk modulus, Young's modulus, and Poisson's ratio are tabulated in books for a variety of materials.
SHEAR STRESS
The forces associated with pressure, with tensile, and with compressive stress all act in a direction perpendicular to the surface at which they are applied. When a board is dragged across the surface of a fluid, it also produces a force parallel to the surface, called the viscous force.
When a force is applied parallel to a solid surface, it is called a shear. The force applied by scissors to a piece of paper is an example of a shear force.
The response of a solid to a shear is similar to the response to a pressure. The sample changes shape, but does not continue to change if the shear stays constant. If the shear is removed, the sample returns to its original shape. It turns out that the word "shape" is advisable, since the volume of the sample does not change in response to a shear stress.
The measure of shear stress is defined as the shear force divided by the area to which it is applied. In order to study the shear stress most simply, the forces are applied in pairs so that the net force on the object is zero, and it is not accelerated. We may imagine a force towards the right applied to the top of a cubic sample, and an equal magnitude force towards the left applied to the bottom of the sample. This stress is called a simple shear.
The simple shear stress has a flaw that we will point out now, and correct later. If no other forces are applied to the sample this pair of forces will produce a torque which gives the object an angular acceleration. We may imagine for now that the experiment is carried out on a table top that prevents this rotation.
For the shear we have imagined, the top of the cube is moved to the
right so that it is no longer directly above the bottom. The vertical sides
of the cube "tilt to the right." The angle that the formerly
vertical sides make with the vertical direction is a good measure of the
distortion caused by the simple shear stress. For small distortion, this
angle (in radians) is equal to the distance that the top shifted, divided
by the height of the cube. We call that ratio the simple
shear strain, 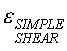
For small angles, the simple shear strain is proportional to the simple
shear stress, 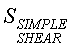
The simple shear elastic constant
is different for each material. It is comparable is magnitude to the bulk
modulus, but smaller. Like the bulk modulus and Young's modulus,
has units of Pa. Like the bulk modulus and Poisson's ratio, the simple
shear modulus,
,
is tabulated for a variety of materials in reference books.
Go forward to next topic.
Return to index.