Arc Length

Suppose a curve is given by continuous functions

$x = f(t), \quad y = g(t), \quad a \le \le b.$

Partition the interval :

$P:\ a = t_0 < t_1 < t_2 < \cdots < t_n = b.$

(Note that different partitions may use different numbers of points, as well as different points.)

Consider a subinterval $[t_k, t_{k + 1}]$ . The corresponding points on the curve are

$(x_k, y_k) = (f(t_k), g(t_k)) \quad\hbox{and}\quad (x_{k + 1}, y_{k + 1}) = (f(t_{k + 1}), g(t_{k + 1})).$

The length of the segment from to $(x_{k + 1}, y_{k + 1})$ is

$\Delta s_k = \sqrt{(x_k - x_{k + 1})^2 + (y_k - y_{k + 1})^2}.$

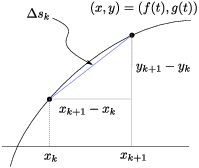
$\hbox{\epsfysize=1.5in \epsffile{arc-length-1.eps}}$

It approximates the length of the curve from to $(x_{k + 1}, y_{k + 1})$ .

For the partition P, the total length of the segments is

$L(P) = \sum_{k = 0}^{n - 1} \Delta s_k.$

Definition. A curve is rectifiable if there is a number M such that for every partition of the interval ,

If a curve is rectifiable, we can define the length of the curve as the least upper bound of taken over all the partitions of the interval.

While you can imagine approximating the length of a curve by taking partitions with larger and larger numbers of points, this definition doesn't give a way of computing the exact length.

If the curve is "well-behaved", we can compute the exact length as follows. Suppose the functions and are differentiable and have continuous derivatives. Apply the Mean Value Theorem to f and to g on a typical subinterval $[t_k, t_{k + 1}]$ . Then there are numbers and such that

$x_{k + 1} - x_k = f'(p_k) (t_{k + 1} - t_k) \quad\hbox{and}\quad y_{k + 1} - y_k = g'(q_k) (t_{k + 1} - t_k).$

Plugging these into the equation for $\Delta s_k$ above, I get

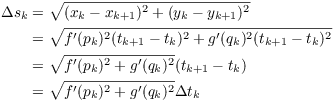
$\eqalign{ \Delta s_k & = \sqrt{(x_k - x_{k + 1})^2 + (y_k - y_{k + 1})^2} \cr & = \sqrt{f'(p_k)^2 (t_{k + 1} - t_k)^2 + g'(q_k)^2 (t_{k + 1} - t_k)^2} \cr & = \sqrt{f'(p_k)^2 + g'(q_k)^2} (t_{k + 1} - t_k) \cr & = \sqrt{f'(p_k)^2 + g'(q_k)^2} \Delta t_k \cr}$

I obtain the sum

$L(P) = \sum_{k = 0}^{n - 1} \Delta s_k = \sum_{k = 0}^{n - 1} \sqrt{f'(p_k)^2 + g'(q_k)^2} \Delta t_k.$

I want to take the limit as the number of subintervals in the partition becomes infinite (or as the length of the subintervals goes to 0). There is a technical point here, and that is that I have two varying quantities and , so this is not an ordinary Riemann sum. In fact, it's possible to show (using a result called Bliss's Theorem) that the Riemann sum produces the expected definite integral:

$L = \int_a^b \sqrt{f'(t)^2 + g'(t)^2}\,dt = \lim_{\Delta t \to 0} \sum_{k = 0}^{n - 1} \sqrt{f'(p_k)^2 + g'(q_k)^2} \Delta t_k.$

This gives the length of the curve. You can also write this in the form

$L = \int_a^b \sqrt{\left(\der x t\right)^2 + \left(\der y t\right)^2}\,dt.$

If the curve is given in the form , we can think of it as parametrized by x (so t becomes x). Since $\der x x = 1$ , the formula is

$L = \int_a^b \sqrt{1 + \left(\der y x\right)^2}\,dx.$

Likewise, if the curve is given in the form , the formula is

$L = \int_a^b \sqrt{1 + \left(\der x y\right)^2}\,dy.$

Example. Find the length of $y = \dfrac{1}{2} x^2$ for $0 \le x \le 1$ .

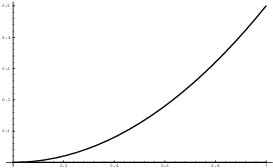
$\hbox{\epsfysize=1.5in \epsffile{arc-length-2.eps}}$

$\der y x = x, \quad\hbox{so}\quad \left(\der y x\right)^2 + 1 = x^2 + 1.$

The length is

$L = \int_0^1 \sqrt{x^2 + 1}\,dx = \left[\dfrac{1}{2}x\sqrt{x^2 + 1} + \dfrac{1}{2} \ln |x + \sqrt{x^2 + 1}|\right]_0^1 = \dfrac{\sqrt{2}}{2} + \dfrac{1}{2} \ln (1 + \sqrt{2}) = 1.14779 \ldots .$

Here's the work for the integral:

$\int \sqrt{x^2 + 1}\,dx = \int \sqrt{(\tan \theta)^2 + 1}(\sec \theta)^2\,d\theta = \int (\sec \theta)^3\,d\theta =$

$\hfil\raise0.5 in\hbox{$\left[x = \tan \theta, \quad dx = (\sec \theta)^2\,d\theta\right]$} \hskip0.5 in \hbox{\epsfysize=1in \epsffile{arc-length-3.eps}}\hfil$

$\dfrac{1}{2}\sec \theta \tan \theta + \dfrac{1}{2}\ln |\sec \theta + \tan \theta| + C = \dfrac{1}{2}x\sqrt{x^2 + 1} + \dfrac{1}{2}\ln |x + \sqrt{x^2 + 1}| + C.\quad\halmos$

Example. Find the length of the curve

$x = e^t \cos 2 t, \quad y = e^t \sin 2 t , \quad 0 \le t \le \dfrac{\pi}{4}.$

$\hbox{\epsfysize=1.5in \epsffile{arc-length-4.eps}}$

$\der x t = -2 e^t \sin 2 t + e^t \cos 2 t, \quad \der y t = 2 e^t \cos 2 t + e^t \sin 2 t,$

$\left(\der x t\right)^2 + \left(\der y t\right)^2 = (-2 e^t \sin 2 t + e^t \cos 2 t)^2 + (2 e^t \cos 2 t + e^t \sin 2 t)^2 =$

$4 e^{2 t}(\sin 2 t)^2 - 4 e^{2 t}\sin 2 t \cos 2 t + e^{2 t}(\cos 2 t)^2 + 4 e^{2 t}(\cos 2 t)^2 + 4 e^{2 t}\sin 2 t \cos 2 t + e^{2 t}(\sin 2 t)^2 =$

$4 e^{2 t}\left[(\sin 2 t)^2 + (\cos 2 t)^2\right] + e^{2 t}\left[(\sin 2 t)^2 + (\cos 2 t)^2\right] = 4 e^{2 t}\cdot 1 + e^{2 t}\cdot 1 = 5 e^{2 t}.$

Hence,

$\sqrt{\left(\der x t\right)^2 + \left(\der y t\right)^2} = \sqrt{5 e^{2 t}} = \sqrt{5}e^t.$

The length is

$L = \int_0^{\pi/4} \sqrt{5}\,e^t\,dt = \sqrt{5} \left[e^t\right]_0^{\pi/4} = \sqrt{5} (e^{\pi/4} - 1) = 2.66825 \ldots .\quad\halmos$

Example. Find the length of $y = \dfrac{x^4}{16} + \dfrac{1}{2 x^2}$ for $1 \le x \le 2$ .

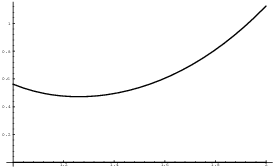
$\hbox{\epsfysize=1.5in \epsffile{arc-length-5.eps}}$

$\der y x = \dfrac{x^3}{4} - \dfrac{1}{x^3}, \quad\hbox{so}\quad \left(\der y x\right)^2 = \left(\dfrac{x^3}{4} - \dfrac{1}{x^3}\right)^2 = \dfrac{x^6}{16} - \dfrac{1}{2} + \dfrac{1}{x^6}.$

The next step is the algebraic trick in this problem:

$\left(\der y x\right)^2 + 1 = \left(\dfrac{x^6}{16} - \dfrac{1}{2} + \dfrac{1}{x^6}\right) + 1 = \dfrac{x^6}{16} + \dfrac{1}{2} + \dfrac{1}{x^6} = \left(\dfrac{x^3}{4} + \dfrac{1}{x^3}\right)^2.$

The idea is that I saw when I found $\left(\der y x\right)^2$ that

$\dfrac{x^6}{16} - \dfrac{1}{2} + \dfrac{1}{x^6} = \left(\dfrac{x^3}{4} - \dfrac{1}{x^3}\right)^2.$

Therefore,

$\dfrac{x^6}{16} + \dfrac{1}{2} + \dfrac{1}{x^6} = \left(\dfrac{x^3}{4} + \dfrac{1}{x^3}\right)^2.$

The only difference is in the sign of the $\dfrac{1}{2}$ . Since the first expression is the square of a binomial with a "-", the second expression must be the square of the same binomial with a "+".

Thus,

$\sqrt{\left(\der y x\right)^2 + 1} = \sqrt{\left(\dfrac{x^3}{4} + \dfrac{1}{x^3}\right)^2} = \dfrac{x^3}{4} + \dfrac{1}{x^3}.$

The length is

$L = \int_1^2 \left(\dfrac{x^3}{4} + \dfrac{1}{x^3}\right)\,dx = \left[\dfrac{x^4}{16} - \dfrac{1}{2 x^2}\right]_1^2 = \dfrac{7}{16} = 0.4375.\quad\halmos$

Contact information

Bruce Ikenaga's Home Page