Infinite Series - Review

Example. Does the series $\displaystyle \sum_{n = 1}^\infty \left(\dfrac{1}{n^2} + 1.01^n\right)$ converge or diverge?

$\displaystyle \sum_{n = 1}^\infty \dfrac{1}{n^2}$ is a p-series with , so it converges. $\displaystyle \sum_{n = 1}^\infty 1.01^n$ is a geometric series with , so it diverges.

Hence, the sum of the two series diverges.

Example. Determine whether the series converges or diverges. If it converges, find its sum.

$\dfrac{7}{16} - \dfrac{7}{64} + \dfrac{7}{256} - \cdots + (-1)^n(7)\left(\dfrac{1}{4}\right)^n + \cdots$

The series is geometric with ratio $-\dfrac{1}{4}$ , so it converges. The sum is

$\dfrac{7}{16} - \dfrac{7}{64} + \dfrac{7}{256} - \cdots + (-1)^n\left(\dfrac{1}{7}\right)\left(\dfrac{1}{4}\right)^n + \cdots = \dfrac{7}{16}\left(1 - \dfrac{1}{4} + \dfrac{1}{16} - \dfrac{1}{64} + \cdots\right) =$

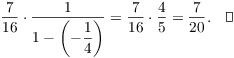
$\dfrac{7}{16}\cdot \dfrac{1}{1 - \left(-\dfrac{1}{4}\right)} = \dfrac{7}{16}\cdot \dfrac{4}{5} = \dfrac{7}{20}.\quad\halmos$

Example. Does the series $\displaystyle \sum_{n = 1}^\infty \dfrac{\sqrt{e^n}}{\root 3 \of {\pi^n}}$ converge or diverge?

$\sum_{n = 1}^\infty \dfrac{\sqrt{e^n}}{\root 3 \of {\pi^n}} = \sum_{n = 1}^\infty \left(\dfrac{\sqrt{e}} {\root 3 \of {\pi}}\right)^n.$

The series is geometric with ratio $\dfrac{\sqrt{e}} {\root 3 \of {\pi}} \approx 1.12572 > 1$ . Therefore, the series diverges.

Example. Does the series $\displaystyle \sum_{n = 1}^\infty \dfrac{4 n^3 + 5}{7 n^2 - 11 n^3}$ converge or diverge?

$\lim_{n \to \infty} \dfrac{4 n^3 + 5}{7 n^2 - 11 n^3} = -\dfrac{4}{11} \ne 0.$

The series diverges by the Zero Limit Test.

Example. Does the series $\displaystyle \sum_{n = 1}^\infty \tan^{-1} \dfrac{42^n}{41^n}$ converge or diverge?

$\dfrac{42^n}{41^n}$ is a geometric sequence with ratio $\dfrac{42}{41} > 1$ , so $\dfrac{42^n}{41^n} \to \infty$ as $n \to \infty$ . Therefore,

$\lim_{n \to \infty} \tan^{-1} \dfrac{42^n}{41^n} = \dfrac{\pi}{2} \ne 0.$

Hence, the series diverges, by the Zero Limit Test.

Example. Does the series $\displaystyle \sum_{n = 2}^\infty \dfrac{1}{n(\ln n)^2}$ converge or diverge?

The terms are positive. The function $f(x) = \dfrac{1}{x(\ln x)^2}$ is continuous for $x \ge 2$ . The derivative is

$f'(x) = -\dfrac{1}{x^2(\ln x)^2} - \dfrac{2}{x^2(\ln x)^3}.$

I have for $x \ge 2$ . Thus, the terms of the series decrease. The hypotheses of the Integral Test are satisfied.

Compute the integral:

$\int_2^\infty \dfrac{1}{x(\ln x)^2}\,dx = \lim_{b \to \infty} \int_2^b \dfrac{1}{x(\ln x)^2}\,dx = \lim_{b \to \infty} \int_{\ln 2}^{\ln b} \dfrac{1}{x\cdot u^2}\cdot x\,du = \lim_{b \to \infty} \int_{\ln 2}^{\ln b} \dfrac{du}{u^2} =$

$\left[u = \ln x, \quad du = \dfrac{dx}{x}, \quad dx = x\,du; \quad x = 2, \quad u = \ln 2; \quad x = b, \quad u = \ln b\right]$

$\lim_{b \to \infty} \left[-\dfrac{1}{u}\right]_{\ln 2}^{\ln b} = \lim_{b \to \infty} \left(-\dfrac{1}{\ln b} + \dfrac{1}{\ln 2}\right) = \dfrac{1}{\ln 2}.$

The integral converges, so the series converges, by the Integral Test.

Example. Does the series $\displaystyle \sum_{n = 1}^\infty \dfrac{1 + e^{-n^3}}{n}$ converge or diverge?

Notice that as $n \to \infty$ , $1 + e^{-n^3}$ decreases to 1. Thus,

$1 + e^{-n^3} \ge 1, \quad\hbox{so}\quad \dfrac{1 + e^{-n^3}}{n} \ge \dfrac{1}{n}.$

$\displaystyle \sum_{n = 1}^\infty \dfrac{1}{n}$ is harmonic, so it diverges. Therefore, the original series diverges by direct comparison.

Example. Does the series $\displaystyle \sum_{n = 1}^\infty \dfrac{\sqrt{n(n + 1)}}{\sqrt{(n + 2)(n + 3)(n + 4)}}$ converge or diverge?

For large n, $\dfrac{\sqrt{n(n + 1)}}{\sqrt{(n + 2)(n + 3)(n + 4)}} \approx \dfrac{\sqrt{n^2}}{\sqrt{n^3}} = \dfrac{1}{n^{1/2}}$ . Do a Limit Comparison:

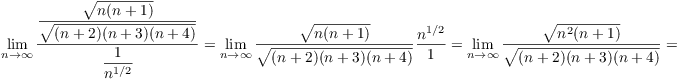
$\lim_{n \to \infty} \dfrac{\dfrac{\sqrt{n(n + 1)}}{\sqrt{(n + 2)(n + 3)(n + 4)}}}{\dfrac{1}{n^{1/2}}} = \lim_{n \to \infty} \dfrac{\sqrt{n(n + 1)}}{\sqrt{(n + 2)(n + 3)(n + 4)}} \dfrac{n^{1/2}}{1} = \lim_{n \to \infty} \dfrac{\sqrt{n^2(n + 1)}}{\sqrt{(n + 2)(n + 3)(n + 4)}} =$

$\sqrt{\lim_{n \to \infty} \dfrac{n^2(n + 1)}{(n + 2)(n + 3)(n + 4)}} = \sqrt{1} = 1.$

The limit is a finite positive number. $\displaystyle \sum_{n = 1}^\infty \dfrac{1}{n^{1/2}}$ diverges, because it's a p-series with $p = \dfrac{1}{2} < 1$ . Therefore, the original series diverges, by Limit Comparison.

Example. Does the series $\displaystyle \sum_{n = 1}^\infty \dfrac{26^n(n!)^3}{(3 n)!}$ converge or diverge?

Apply the Ratio Test:

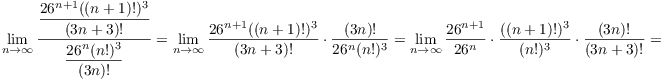
$\lim_{n \to \infty} \dfrac{\dfrac{26^{n+1}((n + 1)!)^3}{(3 n + 3)!}}{\dfrac{26^n(n!)^3}{(3 n)!}} = \lim_{n \to \infty} \dfrac{26^{n+1}((n + 1)!)^3}{(3 n + 3)!} \cdot \dfrac{(3 n)!}{26^n(n!)^3} = \lim_{n \to \infty} \dfrac{26^{n+1}}{26^n} \cdot \dfrac{((n + 1)!)^3}{(n!)^3} \cdot \dfrac{(3 n)!}{(3 n + 3)!} =$

$\lim_{n \to \infty} 26 \cdot \left(\dfrac{(n + 1)!}{n!}\right)^3\cdot \dfrac{(3 n)!}{(3 n + 3)!} =$

$\lim_{n \to \infty} 26 \cdot \left(\dfrac{(1)(2) \cdots (n)(n + 1)}{(1)(2) \cdots (n)}\right)^3 \cdot \dfrac{(1)(2) \cdots (3 n)}{(1)(2) \cdots (3 n)(3 n + 1)(3 n + 2)(3 n + 3)} =$

$\lim_{n \to \infty} 26 \cdot \left(n + 1\right)^3 \cdot \dfrac{1}{(3 n + 1)(3 n + 2)(3 n + 3)} = \lim_{n \to \infty} \dfrac{26(n + 1)^3}{(3 n + 1)(3 n + 2)(3 n + 3)} = \dfrac{26}{27} < 1.$

Therefore, the series converges by the Ratio Test.

Example. Does the series $\displaystyle \sum_{n = 1}^\infty \left(\sin^{-1} \dfrac{1}{n}\right)^n$ converge or diverge?

Apply the Root Test:

$\lim_{n \to \infty} a_n^{1/n} = \lim_{n \to \infty} \left(\sin^{-1} \dfrac{1}{n}\right) = \sin^{-1} 0 = 0 < 1.$

The series converges by the Root Test.

Example. The series $\displaystyle \sum_{k = 1}^\infty (-1)^{k + 1} \dfrac{2}{\root 3 \of {k + 5}}$ converges by the Alternating Series Test.

Find the smallest value of n for which the partial sum $\displaystyle \sum_{k = 1}^n (-1)^{k + 1} \dfrac{2}{\root 3 \of {k + 5}}$ approximates the actual value of the sum to within 0.01.

The error in using $\displaystyle \sum_{k = 1}^n (-1)^{k + 1} \dfrac{2}{\root 3 \of {k + 5}}$ to approximate the actual value of the sum is less than the $(n + 1)^{\rm st}$ term in absolute value, so I want

$\eqalign{ \dfrac{2}{\root 3 \of {(n + 1) + 5}} & < 0.01 \cr \noalign{\vskip2 pt} \dfrac{2}{\root 3 \of {n + 6}} & < 0.01 \cr \noalign{\vskip2 pt} 2 & < 0.01 {\root 3 \of {n + 6}} \cr \noalign{\vskip2 pt} 200 & < {\root 3 \of {n + 6}} \cr 8\,000\,000 & < n + 6 \cr 7\,999\,994 & < n \cr}$

The smallest value of n is $n = 7\,999\,995$ .

Example. Does the series $\displaystyle \sum_{n = 1}^\infty \dfrac{(-1)^{n+1}}{5 n + 2}$ converge absolutely, converge conditionally, or diverge?

Consider the absolute value series $\displaystyle \sum_{n = 1}^\infty \dfrac{1}{5 n + 2}$ . By Limit Comparison,

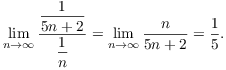
$\lim_{n \to \infty} \dfrac{\dfrac{1}{5 n + 2}}{\dfrac{1}{n}} = \lim_{n \to \infty} \dfrac{n}{5 n + 2} = \dfrac{1}{5}.$

The limit is a finite positive number. $\displaystyle \sum_{n = 1}^\infty \dfrac{1}{n}$ diverges, because it's harmonic. Therefore, $\displaystyle \sum_{n = 1}^\infty \dfrac{1}{5 n + 2}$ diverges by Limit Comparison.

Hence, $\displaystyle \sum_{n = 1}^\infty \dfrac{(-1)^{n+1}}{5 n + 2}$ does not converge absolutely.

Consider the original series $\displaystyle \sum_{n = 1}^\infty \dfrac{(-1)^{n+1}}{5 n + 2}$ . It alternates, and if $f(n) = \dfrac{1}{5 n + 2}$ ,

$f'(n) = -\dfrac{5}{(5 n + 2)^2} < 0 \quad\hbox{for}\quad n \ge 1.$

Hence, the terms decrease in absolute value. Finally,

$\lim_{n \to \infty} \dfrac{1}{5 n + 2} = 0.$

By the Alternating Series Test, $\displaystyle \sum_{n = 1}^\infty \dfrac{(-1)^{n+1}}{5 n + 2}$ converges. Since it converges, but does not converge absolutely, it converges conditionally.

Contact information

Bruce Ikenaga's Home Page