Review Sheet for Test 1

Math 101

These problems are provided to help you study. The presence of a problem on this handout does not imply that there will be a similar problem on the test. And the absence of a topic does not imply that it won't appear on the test.

1. (a) Solve for x: $2 x + 1 = 4 x - 5$ .

(b) Solve for x: $3(x - 2) = 5(x + 1)$ .

(c) Solve for x: $\dfrac{1}{2}x - \dfrac{1}{3}(x - 1) = \dfrac{1}{6}x + 5$ .

(d) Solve for x: $0.35(x + 4) = 0.5(0.7 x + 2.8)$ .

(e) Solve for x: $\dfrac{3}{4} (5 x + 2) = \dfrac{7}{5} - \dfrac{1}{2} x$ .

(f) Solve for x: $-1.13 (2 x - 5) = 0.2 + 0.38 x$ .

2. (a) (Ideal gas law) Solve for T in $pV = nRT$ .

(b) (Area of a trapezoid) Solve for $b_2$ in $A = \dfrac{1}{2}h(b_1 + b_2)$ .

(c) Solve for c in $a b c + 2 b c - 3 a b P = 0$ .

(d) Solve for k in $ak = b(k + 1)$ .

3. (a) Solve $3 x + 5 > 4(2 x - 1)$ . Write your answer using inequality notation and interval notation, and draw a picture of the solution set.

(b) Solve $-3 x - 4 \le -8(x + 5)$ . Write your answer using inequality notation and interval notation, and draw a picture of the solution set.

(c) Solve $0.5(6 x - 4) < 3 x - 10$ . Write your answer using inequality notation and interval notation, and draw a picture of the solution set.

(d) Solve $-4 < 2(x + 1) < 10$ . Write your answer using inequality notation and interval notation, and draw a picture of the solution set.

(e) Solve $\dfrac{2}{3} x - \dfrac{1}{4} < \dfrac{5}{6}(x + 1)$ . Write your answer using inequality notation, and draw a picture of the solution set.

4. (a) Solve for x: $|x - 10| = 5$ .

(b) Solve for x: $5 + |x + 7| = 13$ .

(c) Solve for x: $|x - 4| = -6$ .

(d) Solve for x: $|2 x + 3| = 17$ .

5. (a) Solve for x: $|x - 1| < 4$ .

(b) Solve for x: $|x + 6| \ge 3$ .

(c) Solve for x: $|x - 17| < -2$ .

(d) Solve for x: $|2 x - 5| > 13$ .

(e) Solve for x: $|-x - 6| \le 1$ .

6. Find the slope of the line passing through $(1,3)$ and $(-2,-5)$ .

7. Find the slope and y-intercept of the line $3 x - 6y = 18$ .

8. Find the x and y-intercepts of the line $7 x - 2 y = 21$ . Then use them to graph the line.

9. Find the equation of the line which passes through the point $(2,-3)$ and is parallel to the line $y = -17 x + 21$ .

10. Find the equation of the line which is perpendicular to the line $8 x - 2y = 5$ and which has y-intercept 13.

11. Find the equation of the line with slope 7 which passes through the point $(1,-5)$ .

12. Find the equation of the line which passes through the points $(1,-3)$ and $(2,5)$ .

13. Find the equation of the line with slope 5 and y-intercept 42.

14. What is the x-intercept of the line $y = 7 x - 28$ ?

15. Find the equation of the line which is parallel to $2 x + 7y + 8 = 0$ and passes through the point $(4,-9)$ .

16. Find the slope of a line which is perpendicular to the line containing the points $(2,3)$ and $(0,-8)$ .

17. Find the equation of the vertical line which passes through the point $(17,-33)$ .

18. Find the equation of the line whose graph is shown below.

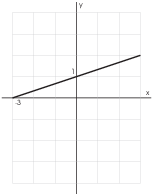
$$\hbox{\epsfysize=1.75in \epsffile{rev1-4.eps}}$$

19. Graph the line $-3 x + 2y = 6$ by finding the x-intercept and y-intercept.

20. What is the x-intercept of the line $y = 11$ ?

21. Find the point or points of intersection of the lines

$$3 x + 2y = 4 \quad\hbox{and}\quad 2 x + y = -7.$$

22. Solve the system of equations

$$\eqalign{5 x + 2y & = 8 \cr 2 x + y & = 1 \cr}$$

23. Solve the system of equations

$$\eqalign{3 x - 2y & = 7 \cr -9 x + 6y & = 10 \cr}$$

24. Solve the system of equations

$$\eqalign{2 x + 5y & = 7 \cr 0.4 x + y & = 1.4 \cr}$$

25. Solve the system of equations

$$\eqalign{ 2 x + 3 y & = 11 \cr 3 x - 2 y & = -16 \cr}$$

26. Solve the system of equations

$$\eqalign{ 3 x + 5 y & = 1 \cr 4 x - y & = 11 \cr}$$

27. Solve the system of equations

$$\eqalign{2 x - 3y & = 11 \cr -4 x + 6y & = -25 \cr}$$

28. Five more than three times a number is equal to 19 less than the number. Find the number.

29. The perimeter of a rectangle is 50 meters. The length is 1 meter more than twice the width. Find the dimensions.

30. Calvin drives down a long straight road in his 1978 Chevette at a constant speed of 30 miles an hour. Phoebe starts out 50 miles behind him, and follows him at 70 miles an hour in her Porsche. How long will it take before Phoebe catches up to Calvin?

31. Leopold drives 6 miles per hour faster than Molly. If they start at the same point and drive in opposite directions for 4 hours, they will be 272 miles apart. What is Molly's speed?

32. Phoebe invests $2000 in two accounts. One pays 6% simple interest, while the other pays 8% simple interest. At the end of a year, she has earned $128 in interest. How much was invested in each account?

33. After one interest period, the interest on a $700 investment is $3 greater than the interest on a $500 investment. The $700 is invested at a rate $0.6%$ higher than the rate for the $500 investment. Find the interest rate for each investment.

34. The sum of two numbers is 153. The second number is 5 more than 3 times the first number. Find the numbers.

35. Calvin Butterball has $4.00 in dimes and nickels. The number of dimes is 5 less than twice the number of nickels. Find the number of dimes and the number of nickels.

36. Sarevok mixes an alloy containing $18%$ silver with an alloy containing $30%$ silver to make 50 pounds of an alloy with $26.4%$ silver. How many pounds of each kind of alloy did he use?

37. Silas Hogwinder has some 13-cent stamps, some 17-cent stamps, and some 40-cent stamps. The number of 13-cent stamps is 2 less than the number of 17-cent stamps. The number of 40-cent stamps is 1 more than twice the number of 17-cent stamps. The total value of the stamps is $13.34. Find the number of each type of stamp that Silas has.

38. How many gallons of a $40%$ alcohol solution and a $30%$ alcohol solution must be mixed together to make 50 gallons of a $32%$ alcohol solution?

39. How many pounds of dried fruit worth $7 per pound must be mixed with 4 pounds of peppermint ketchup worth $3.50 per pound to make a mixture worth $5 per pound?

40. Bonzo divides $1000 up between two accounts. The first account pays $6%$ annual interest, while the second pays $8%$ annual interest. After one year, the interest earned by the $8%$ account was $31 more than the interest earned by the $6%$ account. Find the amounts that were invested in the two accounts.

41. Gordon Freeman goes on a car trip of 345 miles that takes a total of 6 hours. He averages 54 miles per hour for the first part of the trip; after a rest stop, he continue his drive and averages 60 miles per hour for the second part of the trip. How long were the two parts of the trip?

Solutions to the Review Sheet for Test 1

1. (a) Solve for x: $2 x + 1 = 4 x - 5$ .

(b) Solve for x: $3(x - 2) = 5(x + 1)$ .

(c) Solve for x: $\dfrac{1}{2} x - \dfrac{1}{3} (x - 1) = \dfrac{1}{6} x + 5$ .

(d) Solve for x: $0.35 (x + 4) = 0.5 (0.7 x + 2.8)$ .

(e) Solve for x: $\dfrac{3}{4} (5 x + 2) = \dfrac{7}{5} - \dfrac{1}{2} x$ .

(f) Solve for x: $-1.13 (2 x - 5) = 0.2 + 0.38 x$ .

(a)

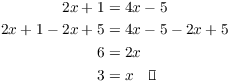
$$\eqalign{ 2 x + 1 & = 4 x - 5 \cr 2 x + 1 - 2 x + 5 & = 4 x - 5 - 2 x + 5 \cr 6 & = 2 x \cr 3 & = x \quad\halmos \cr}$$

(b)

$$\eqalign{ 3(x - 2) & = 5(x + 1) \cr 3 x - 6 & = 5 x + 5 \cr 3 x - 6 - 3 x - 5 & = 5 x + 5 - 3 x - 5 \cr -11 & = 2 x \cr \noalign{\vskip2pt} -\dfrac{11}{2} & = x \quad\halmos \cr}$$

(c)

$$\eqalign{ \dfrac{1}{2} x - \dfrac{1}{3} (x - 1) & = \dfrac{1}{6} x + 5 \cr \noalign{\vskip2pt} 6 \cdot \left[\dfrac{1}{2} x - \dfrac{1}{3} (x - 1)\right] & = 6 \cdot \left(\dfrac{1}{6} x + 5\right) \cr \noalign{\vskip2pt} 6 \cdot \dfrac{1}{2} x - 6 \cdot \dfrac{1}{3} (x - 1) & = 6 \cdot \dfrac{1}{6} x + 6 \cdot 5 \cr \noalign{\vskip2pt} 3 x - 2 (x - 1) & = x + 30 \cr 3 x - 2 x + 2 & = x + 30 \cr x + 2 & = x + 30 \cr x + 2 - x & = x + 30 - x \cr 2 & = 30 \cr}$$

The last equation "$2 = 30$ " is a contradiction. Therefore, the original equation has no solutions.

(d)

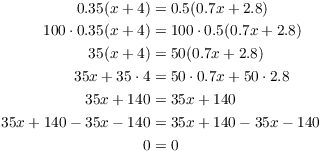
$$\eqalign{ 0.35 (x + 4) & = 0.5 (0.7 x + 2.8) \cr 100 \cdot 0.35 (x + 4) & = 100 \cdot 0.5 (0.7 x + 2.8) \cr 35 (x + 4) & = 50 (0.7 x + 2.8) \cr 35 x + 35 \cdot 4 & = 50 \cdot 0.7 x + 50 \cdot 2.8 \cr 35 x + 140 & = 35 x + 140 \cr 35 x + 140 - 35 x - 140 & = 35 x + 140 - 35 x - 140 \cr 0 & = 0 \cr}$$

The last equation is an identity (an equation that is true for all x --- and there are no x's!). Therefore, the original equation is true for all x. (You could also say the solution is "all real numbers".)

(e)

$$\eqalign{ \dfrac{3}{4} (5 x + 2) & = \dfrac{7}{5} - \dfrac{1}{2} x \cr \noalign{\vskip2pt} 20 \cdot \dfrac{3}{4} (5 x + 2) & = 20 \cdot \left(\dfrac{7}{5} - \dfrac{1}{2} x\right) \cr \noalign{\vskip2pt} 20 \cdot \dfrac{3}{4} (5 x + 2) & = 20 \cdot \dfrac{7}{5} - 20 \cdot \dfrac{1}{2} x \cr \noalign{\vskip2pt} 15 (5 x + 2) & = 28 - 10 x \cr 75 x + 30 & = 28 - 10 x \cr 75 x + 30 - 30 + 10 x & = 28 - 10 x - 30 + 10 x \cr 85 x & = -2 \cr \noalign{\vskip2pt} x & = -\dfrac{2}{85} \quad\halmos \cr}$$

(f)

$$\eqalign{ -1.13 (2 x - 5) & = 0.2 + 0.38 x \cr 100 \cdot -1.13 (2 x - 5) & = 100 \cdot \left(0.2 + 0.38 x\right) \cr 100 \cdot -1.13 (2 x - 5) & = 100 \cdot 0.2 + 100 \cdot 0.38 x \cr -113 (2 x - 5) & = 20 + 38 x \cr -226 x + 565 & = 20 + 38 x \cr -226 x + 565 - 565 - 38 x & = 20 + 38 x - 565 - 38 x \cr -264 x & = -545 \cr \noalign{\vskip2pt} x & = \dfrac{565}{264} \quad\halmos \cr}$$

2. (a) (Ideal gas law) Solve for T in $pV = nRT$ .

(b) (Area of a trapezoid) Solve for $b_2$ in $A = \dfrac{1}{2}h (b_1 + b_2)$ .

(c) Solve for c in $a b c + 2 b c - 3 a b P = 0$ .

(d) Solve for k in $a k = b(k + 1)$ .

(a)

$$\eqalign{ p V & = n R T \cr \dfrac{p V}{n R} & = \dfrac{n R T}{n R} \cr \dfrac{p V}{n R} & = T \quad\halmos \cr}$$

(b)

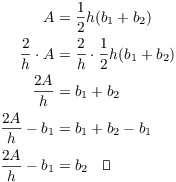
$$\eqalign{ A & = \dfrac{1}{2} h (b_1 + b_2) \cr \dfrac{2}{h}\cdot A & = \dfrac{2}{h}\cdot \dfrac{1}{2} h (b_1 + b_2) \cr \dfrac{2 A}{h} & = b_1 + b_2 \cr \dfrac{2 A}{h} - b_1 & = b_1 + b_2 - b_1 \cr \dfrac{2 A}{h} - b_1 & = b_2 \quad\halmos \cr}$$

(c)

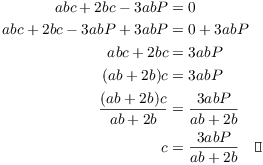
$$\eqalign{a b c + 2 b c - 3 a b P & = 0 \cr a b c + 2 b c - 3 a b P + 3 a b P & = 0 + 3 a b P \cr a b c + 2 b c & = 3 a b P \cr (a b + 2 b) c & = 3 a b P \cr \dfrac{(a b + 2 b) c}{a b + 2 b} & = \dfrac{3 a b P}{a b + 2 b} \cr c & = \dfrac{3 a b P}{a b + 2 b} \quad\halmos \cr}$$

(d)

$$\eqalign{ a k & = b (k + 1) \cr a k & = b k + b \cr a k - b k & = b \cr k (a - b) & = b \cr \dfrac{k (a - b)}{a - b} & = \dfrac{b}{a - b} \cr k & = \dfrac{b}{a - b} \quad\halmos \cr}$$

3. (a) Solve $3 x + 5 > 4(2 x - 1)$ . Write your answer using inequality notation and interval notation, and draw a picture of the solution set.

(b) Solve $-3 x - 4 \le -8(x + 5)$ . Write your answer using inequality notation and interval notation, and draw a picture of the solution set.

(c) Solve $0.5(6 x - 4) < 3 x - 10$ . Write your answer using inequality notation and interval notation, and draw a picture of the solution set.

(d) Solve $-4 < 2(x + 1) < 10$ . Write your answer using inequality notation, and draw a picture of the solution set.

(e) Solve $\dfrac{2}{3} x - \dfrac{1}{4} < \dfrac{5}{6}(x + 1)$ . Write your answer using inequality notation, and draw a picture of the solution set.

(a)

$$\matrix{& 3 x & + & 5 & > & & 4(2 x - 1) & \cr & 3 x & + & 5 & > & 8 x & - & 4 \cr - & 3 x & & & & 3 x & & \cr \noalign{\vskip2pt\hrule\vskip2pt} & & & 5 & > & 5 x & - & 4 \cr + & & & 4 & & & & 4 \cr \noalign{\vskip2pt\hrule\vskip2pt} & & & 9 & > & 5 x & & \cr \div & & & 5 & & 5 & & \cr \noalign{\vskip2pt\hrule\vskip2pt} & & & \dfrac{9}{5} & > & x & & \cr}$$

The solution is $\left(-\infty, \dfrac{9}{5}\right)$ .

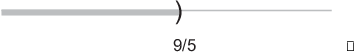
$$\hbox{\epsfxsize=3in \epsffile{rev1-8a-brackets.eps}}\quad\halmos$$

(b)

$$\matrix{& -3 x & - & 4 & \le & & -8(x + 5) & \cr & -3 x & - & 4 & \le & -8 x & - & 40 \cr + & 3 x & & & & 3 x & & \cr \noalign{\vskip2pt\hrule\vskip2pt} & & & -4 & \le & -5 x & - & 40 \cr + & & & 40 & & & & 40 \cr \noalign{\vskip2pt\hrule\vskip2pt} & & & 36 & \le & -5 x & & \cr \div & & & -5 & & -5 & & \cr \noalign{\vskip2pt\hrule\vskip2pt} & & & -\dfrac{36}{5} & \ge & x & & \cr}$$

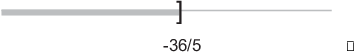
Notice that the inequality "flipped over" when I divided by the negative number -5. The solution is $\left(-\infty, -\dfrac{36}{5}\right]$ .

$$\hbox{\epsfxsize=3in \epsffile{rev1-8b-brackets.eps}}\quad\halmos$$

(c)

$$\matrix{& & 0.5(6 x - 4) & & < & 3 x & - & 10 \cr & 3 x & - & 2 & < & 3 x & - & 10 \cr - & 3 x & & & & 3 x & & \cr \noalign{\vskip2pt\hrule\vskip2pt} & & & -2 & < & & & -10 \cr}$$

The last statement "$-2 < -10$ " is false. Therefore, the original inequality has no solutions.

(d)

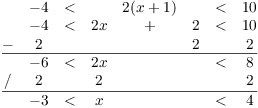
$$\matrix{& -4 & < & & 2(x + 1) & & < & 10 \cr & -4 & < & 2 x & + & 2 & < & 10 \cr - & 2 & & & & 2 & & 2 \cr \noalign{\vskip2pt\hrule\vskip2pt} & -6 & < & 2 x & & & < & 8 \cr / & 2 & & 2 & & & & 2 \cr \noalign{\vskip2pt\hrule\vskip2pt} & -3 & < & x & & & < & 4 \cr}$$

The solution is $(-3, 4)$ .

$$\hbox{\epsfxsize=3in \epsffile{rev1-8d-brackets.eps}}\quad\halmos$$

(e)

$$\eqalign{ \dfrac{2}{3} x - \dfrac{1}{4} & < \dfrac{5}{6}(x + 1) \cr \noalign{\vskip2pt} 12 \cdot \dfrac{2}{3} x - 12 \cdot \dfrac{1}{4} & < 12 \cdot \dfrac{5}{6}(x + 1) \cr \noalign{\vskip2pt} 8 x - 3 & < 10(x + 1) \cr 8 x - 3 & < 10 x + 10 \cr 8 x - 3 - 8 x - 10 & < 10 x + 10 - 8 x - 10 \cr -13 & < 2 x \cr \noalign{\vskip2pt} -\dfrac{13}{2} & < x \cr}$$

The solution is $\left(-\dfrac{13}{2}, \infty\right)$ .

$$\hbox{\epsfxsize=3in \epsffile{rev1-8e-brackets.eps}}\quad\halmos$$

4. (a) Solve for x: $|x - 10| = 5$ .

(b) Solve for x: $5 + |x + 7| = 13$ .

(c) Solve for x: $|x - 4| = -6$ .

(d) Solve for x: $|2 x + 3| = 17$ .

(a)

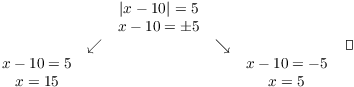
$$\matrix{ & & |x - 10| = 5 & & \cr & & x - 10 = \pm 5 & & \cr & \swarrow & & \searrow & \cr x - 10 = 5 & & & & x - 10 = -5 \cr x = 15 & & & & x = 5 \cr}\quad\halmos$$

(b)

$$\matrix{ & & 5 + |x + 7| = 13 & & \cr & & |x + 7| = 8 & & \cr & & x + 7 = \pm 8 & & \cr & \swarrow & & \searrow & \cr x + 7 = 8 & & & & x + 7 = -8 \cr x = 1 & & & & x = -15 \cr} \quad\halmos$$

(c) The absolute value of $x - 4$ must be greater than or equal to 0; it can't be negative, so it can't be equal to -6. Therefore, there are no solutions.

(d)

$$\matrix{ & & |2 x + 3| = 17 & & \cr & \swarrow & & \searrow & \cr 2 x + 3 = 17 & & & & 2 x + 3 = -17 \cr 2 x = 14 & & & & 2 x = -20 \cr x = 7 & & & & x = -10 \cr} \quad\halmos$$

5. (a) Solve for x: $|x - 1| < 4$ .

(b) Solve for x: $|x + 6| \ge 3$ .

(c) Solve for x: $|x - 17| < -2$ .

(d) Solve for x: $|2 x - 5| > 13$ .

(e) Solve for x: $|-x - 6| \le 1$ .

(a) Since the "$|x - 1|$ " is on the "small" side of the "$<$ ", the solution will be the inner interval:

$$\hbox{\epsfxsize=3in \epsffile{rev1-10a-1.eps}}$$

Find the break points by solving the corresponding equality:

$$\matrix{ & & |x - 1| = 4 & & \cr & & x - 1 = \pm 4 & & \cr & \swarrow & & \searrow & \cr x - 1 = 4 & & & & x - 1 = -4 \cr x = 5 & & & & x = -3 \cr}$$

$$\hbox{\epsfxsize=3in \epsffile{rev1-10a-2.eps}}$$

Write down the inequality for the shaded region. The solution is $-3 < x < 5$ , or $(-3, 5)$ .

(b) Since the "$|x + 6|$ " is on the "big" side of the "$\ge$ ", the solution will be the outer intervals:

$$\hbox{\epsfxsize=3in \epsffile{rev1-10b-1.eps}}$$

Find the break points by solving the corresponding equality:

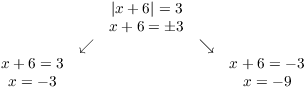
$$\matrix{ & & |x + 6| = 3 & & \cr & & x + 6 = \pm 3 & & \cr & \swarrow & & \searrow & \cr x + 6 = 3 & & & & x + 6 = -3 \cr x = -3 & & & & x = -9 \cr}$$

$$\hbox{\epsfxsize=3in \epsffile{rev1-10b-2.eps}}$$

Write down the inequality for the shaded region. The solution is $x \le -9$ or $-3 \le x$ . In interval notation, this is $(-\infty, -9) \cup (-3, \infty)$ .

(c) The absolute value of $x - 17$ is nonegative, so it can't be less than -2. Therefore, there are no solutions.

(d) Since the "$|2 x - 5|$ " is on the "big" side of the "$>$ ", the solution will be the outer intervals:

$$\hbox{\epsfxsize=3in \epsffile{rev1-10d-1.eps}}$$

Find the break points by solving the corresponding equality:

$$\matrix{ & & |2 x - 5| = 13 & & \cr & & 2 x - 5 = \pm 13 & & \cr & \swarrow & & \searrow & \cr 2 x - 5 = 13 & & & & 2 x - 5 = -13 \cr 2 x = 18 & & & & 2 x = -8 \cr x = 9 & & & & x = -4 \cr}$$

$$\hbox{\epsfxsize=3in \epsffile{rev1-10d-2.eps}}$$

Write down the inequality for the shaded region. The solution is $x < -4$ or $9 < x$ . In interval notation, this is $(\infty, -4) \cup (9, \infty)$ .

(e) Since the "$|-x - 6|$ " is on the "small" side of the "$\le$ ", the solution will be the inner interval:

$$\hbox{\epsfxsize=3in \epsffile{rev1-10e-1.eps}}$$

Find the break points by solving the corresponding equality:

$$\matrix{ & & |-x - 6| = 1 & & \cr & & -x - 6 = \pm 1 & & \cr & \swarrow & & \searrow & \cr -x - 6 = 1 & & & & -x - 6 = -1 \cr -x = 7 & & & & -x = 5 \cr x = -7 & & & & x = -5 \cr}$$

$$\hbox{\epsfxsize=3in \epsffile{rev1-10e-2.eps}}$$

Write down the inequality for the shaded region. The solution is $-7 \le x \le -5$ . In interval notation, this is $[-7, -5]$ .

6. Find the slope of the line passing through $(1,3)$ and $(-2,-5)$ .

$$m = \dfrac{3 - (-5)}{1 - (-2)} = \dfrac{8}{3}.\quad\halmos$$

7. Find the slope and y-intercept of the line $3 x - 6y = 18$ .

Put the equation into slope-intercept form by solving for y:

$$\matrix{& 3 x & - & 6y & = & & & 18 \cr - & 3 x & & & & 3 x & & \cr \noalign{\vskip2pt\hrule\vskip2pt} & & & -6y & = & -3 x & + & 18 \cr / & & & -6 & & -6 & & -6 \cr \noalign{\vskip2pt\hrule\vskip2pt} & & & y & = & \dfrac{1}{2}x & - & 3 \cr}$$

The slope is $\dfrac{1}{2}$ and the y-intercept is -3.

8. Find the x and y-intercepts of the line $7 x - 2y = 21$ . Then use them to graph the line.

Setting $x = 0$ , I get $-2y = 21$ , so $y = -\dfrac{21}{2}$ . The y-intercept is $-\dfrac{21}{2}$ .

Setting $y = 0$ , I get $7 x = 21$ , so $x = 3$ . The x-intercept is 3.

$$\hbox{\epsfysize=1.75in \epsffile{rev1-6c.eps}}\quad\halmos$$

9. Find the equation of the line which passes through the point $(2, -3)$ and is parallel to the line $y = -17 x + 21$ .

The line $y = -17 x + 21$ has slope -17. The line I want is parallel to this line, so it also has slope -17. It passes through the point $(2,-3)$ , so the line is

$$y + 3 = -17(x - 2) \quad\hbox{or}\quad y = -17 x + 31.\quad\halmos$$

10. Find the equation of the line which is perpendicular to the line $8 x - 2y = 5$ and which has y-intercept 13.

Solve $8 x - 2y = 5$ for y to find the slope:

$$\matrix{& 8 x & - & 2y & = & & & 5 \cr - & 8 x & & & & 8 x & & \cr \noalign{\vskip2pt\hrule\vskip2pt} & & & -2y & = & -8 x & + & 5 \cr / & & & -2 & & -2 & & -2 \cr \noalign{\vskip2pt\hrule\vskip2pt} & & & y & = & 4 x & - & \dfrac{5}{2} \cr}$$

The given line has slope 4. The line I want is perpendicular to the given line, so it has slope $-\dfrac{1}{4}$ . Since the line I want has y-intercept 13, its equation is

$$y = -\dfrac{1}{4}x + 13.\quad\halmos$$

11. Find the equation of the line with slope 7 which passes through the point $(1,-5)$ .

$$7(x - 1) = y + 5.\quad\halmos$$

12. Find the equation of the line which passes through the points $(1,-3)$ and $(2,5)$ .

The slope is $m = \dfrac{5 - (-3)}{2 - 1} = 8$ , so the line is

$$8(x - 1) = y + 3.\quad\halmos$$

13. Find the equation of the line with slope 5 and y-intercept 42.

$$y = 5 x + 42.\quad\halmos$$

14. What is the x-intercept of the line $y = 7 x - 28$ ?

Set $y = 0$ :

$$0 = y = 7 x - 28, \quad 7 x = 28, \quad x = 4.\quad\halmos$$

15. Find the equation of the line which is parallel to $2 x + 7y + 8 = 0$ and passes through the point $(4,-9)$ .

First, put the given line into slope-intercept form:

$$\matrix{& 2 x & + & 7y & + & 8 & = & 0 \cr - & & & 7y & & & & 7y \cr \noalign{\vskip2pt\hrule\vskip2pt} & 2 x & & & + & 8 & = & -7y \cr \div & -7 & & & & -7 & & -7 \cr \noalign{\vskip2pt\hrule\vskip2pt} & -\dfrac{2}{7}x & & & - & \dfrac{8}{7} & = & y \cr}$$

The given line has slope $-\dfrac{2}{7}$ .

Since the line I want is parallel to the given line, the line I want also has slope $-\dfrac{2}{7}$ .

The line I want passes through the point $(4,-9)$ .

Therefore, the line is

$$y + 9 = -\dfrac{2}{7}(x - 4).\quad\halmos$$

16. Find the slope of a line which is perpendicular to the line containing the points $(2, 3)$ and $(0, -8)$ .

The line containing the points $(2, 3)$ and $(0, -8)$ has slope

$$\dfrac{3 - (-8)}{2 - 0} = \dfrac{11}{2}.$$

A line perpendicular to this line has slope equal to the negative reciprocal of $\dfrac{11}{2}$ , which is $-\dfrac{2}{11}$ .

17. Find the equation of the vertical line which passes through the point $(17,-33)$ .

A vertical line has an equation of the form $x = (\hbox{a number})$ . In this case, the number is the x-coordinate of $(17,-33)$ --- that is, 17. So the vertical line which passes through the point $(17,-33)$ is $x = 17$ .

18. Find the equation of the line whose graph is shown below.

$$\hbox{\epsfysize=1.75in \epsffile{rev1-4.eps}}$$

The y-intercept of the line is $b = 1$ .

The line rises 1 unit for every 3 units it moves to the right. Therefore, the slope is $\dfrac{1}{3}$ . (You can also find the slope by picking two points on the line --- for example, $(-3,0)$ and $(0,1)$ --- and applying the slope formula.)

Therefore, the line is

$$y = \dfrac{1}{3}x + 1.\quad\halmos$$

19. Graph the line $-3 x + 2y = 6$ by finding the x-intercept and y-intercept.

When $x = 0$ , I get $2y = 6$ , or $y = 3$ .

When $y = 0$ , I get $-3 x = 6$ , or $x = -2$ .

$$\hbox{\epsfysize=1.75in \epsffile{rev1-5.eps}}\quad\halmos$$

20. What is the x-intercept of the line $y = 11$ ?

The line $y = 11$ is a horizontal line 11 units above the x-axis. Therefore, it doesn't intersect the x-axis, and there is no x-intercept.

21. Find the point or points of intersection of the lines

$$3 x + 2y = 4 \quad\hbox{and}\quad 2 x + y = -7.$$

Solve the equations simultaneously. The second equation gives $y = -2 x - 7$ , so plugging this into the first gives

$$3 x + 2(-2 x - 7) = 4, \quad 3 x - 4 x - 14 = 4, \quad -x - 14 = 4, \quad -x = 18, \quad x = -18.$$

Hence, $y = -2(-18) - 7 = 29$ . The point of intersection is $(-18, 29)$ .

22. Solve the system of equations

$$\eqalign{ 5 x + 2y & = 8 \cr 2 x + y & = 1 \cr}$$

Multiply the second equation by 2 and subtract it from the first:

$$\matrix{ 5 x & + & 2y & = & 8 \cr 4 x & + & 2y & = & 2 \cr \noalign{\vskip2pt\hrule\vskip2pt} x & & & = & 6 \cr}$$

Multiply the first equation by 2, the second equation by 5, and subtract the resulting equations:

$$\matrix{ 10 x & + & 4y & = & 16 \cr 10 x & + & 5y & = & 5 \cr \noalign{\vskip2pt\hrule\vskip2pt} & & -y & = & 11 \cr}$$

Multiplying this by -1, I get $y = -11$ . The solution is $x = 6$ and $y = -11$ .

23. Solve the system of equations

$$\eqalign{ 3 x - 2y & = 7 \cr -9 x + 6y & = 10 \cr}$$

Multiply the first equation by 3 and add the second equation:

$$\matrix{ 9 x & - & 6y & = & 21 \cr -9 x & + & 6y & = & 10 \cr \noalign{\vskip2pt\hrule\vskip2pt} & & 0 & = & 31 \cr}$$

This is a contradiction. Therefore, the system has no solutions.

24. Solve the system of equations

$$\eqalign{ 2 x + 5y & = 7 \cr 0.4 x + y & = 1.4 \cr}$$

Multiply the second equation by 5 and subtract it from the first equation:

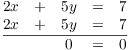
$$\matrix{ 2 x & + & 5y & = & 7 \cr 2 x & + & 5y & = & 7 \cr \noalign{\vskip2pt\hrule\vskip2pt} & & 0 & = & 0 \cr}$$

This is an identity. Hence, the system has infinitely many solutions.

Note: The answer is not "all real numbers". There are infinitely many pairs of numbers that solve the system, but not every pair of numbers is a solution. For example, $x = 0$ and $y = 0$ is not a solution.

25. Solve the system of equations

$$\eqalign{ 2 x + 3 y & = 11 \cr 3 x - 2 y & = -16 \cr}$$

Multiply the first equation by 2 and the second by 3, then add the resulting equations:

$$\eqalign{ 4 x + 6 y & = 22 \cr 9 x - 6 y & = - 48 \cr \noalign{\vskip2pt \hrule \vskip2pt} 13 x & = - 26 \cr x & = -2 \cr}$$

Multiply the first equation by 3 and the second by 2, then subtract the equations:

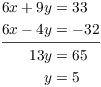
$$\eqalign{ 6 x + 9 y & = 33 \cr 6 x - 4 y & = -32 \cr \noalign{\vskip2pt \hrule \vskip2pt} 13 y & = 65 \cr y & = 5 \cr}$$

The solution is $x = -2$ and $y = 5$ .

26. Solve the system of equations

$$\eqalign{ 3 x + 5 y & = 1 \cr 4 x - y & = 11 \cr}$$

Multiply the first equation by 4, multiply the second equation by 3, then subtract the equations:

$$\eqalign{ 12 x + 20 y & = 4 \cr 12 x - 3 y & = 33 \cr \noalign{\vskip2pt \hrule \vskip2pt} 23 y & = -29 \cr \noalign{\vskip2pt} y & = -\dfrac{29}{23} \cr}$$

Multiply the second equation by 5 and add the first equation:

$$\eqalign{ 3 x + 5 y & = 1 \cr 20 x - 5 y & = 55 \cr \noalign{\vskip2pt \hrule \vskip2pt} 23 x & = 56 \cr \noalign{\vskip2pt} x & = \dfrac{56}{23} \cr}$$

The solution is $x = \dfrac{56}{23}$ and $y = -\dfrac{29}{23}$ .

27. Solve the system of equations

$$\eqalign{ 2 x - 3y & = 11 \cr -4 x + 6y & = -25 \cr}$$

Multiply the first equation by 2 and add it to the second equation:

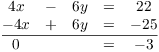
$$\matrix{4 x & - & 6y & = & 22 \cr -4 x & + & 6y & = & -25 \cr \noalign{\vskip2pt\hrule\vskip2pt} 0 & & & = & -3 \cr}$$

The last equation is a contradiction. Therefore, there are no solutions.

28. Five more than three times a number is equal to 19 less than the number. Find the number.

Let x be the number. The first sentence says in symbols:

$$5 + 3 x = x - 19.$$

(Notice that "19 less than the number" translates to $x - 19$ , not $19 - x$ .) Solve the equation for x:

$$\eqalign{ 5 + 3 x & = x - 19 \cr 5 + 3 x - x - 5 & = x - 19 - x - 5 \cr 2 x & = -24 \cr x & = -12 \quad\halmos \cr}$$

29. The perimeter of a rectangle is 50 meters. The length is 1 meter more than twice the width. Find the dimensions.

Let L be the length and let W be the width.

The perimeter is 50 meters: $50 = 2L + 2W$ .

The length is 1 meter more than twice the width: $L = 1 + 2W$ .

Plug $L = 1 + 2W$ into $50 = 2L + 2W$ and simplify:

$$50 = 2(1 + 2W) + 2W, \quad 50 = 2 + 4W + 2W, \quad 50 = 2 + 6W.$$

Solve for W:

$$\matrix{& 50 & = & 2 & + & 6W \cr - & 2 & & 2 & & \cr \noalign{\vskip2pt\hrule\vskip2pt} & 48 & = & & & 6W \cr \div & 6 & & & & 6 \cr \noalign{\vskip2pt\hrule\vskip2pt} & 8 & = & & & W \cr}$$

$W = 8$ gives $L = 1 + 2W = 1 + 2\cdot 8 = 17$ . The width is 8 meters and the length is 17 meters.

30. Calvin drives down a long straight road in his 1978 Chevette at a constant speed of 30 miles an hour. Phoebe starts out 50 miles behind him, and follows him at 70 miles an hour in her Porsche. How long will it take before Phoebe catches up to Calvin?

Let t be the time (in hours) that Calvin travels before Phoebe catches up with him.

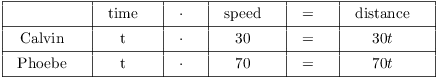
$$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & & & time & & $\cdot$ & & speed & & $=$ & & distance & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & Calvin & & t & & $\cdot$ & & 30 & & $=$ & & $30t$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & Phoebe & & t & & $\cdot$ & & 70 & & $=$ & & $70t$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} }} $$

Since Phoebe started 50 miles behind Calvin, she must travel 50 miles more than Calvin:

$$30t + 50 = 70t.$$

Solve for t:

$$\eqalign{ 30t + 50 & = 70t \cr 50 & = 40t \cr \dfrac{5}{4} & = t \cr}$$

Now $\dfrac{5}{4} = 1.25$ , so it takes Phoebe 1.25 hours (1 hour and 15 minutes) to catch up.

31. Leopold drives 6 miles per hour faster than Molly. If they start at the same point and drive in opposite directions for 4 hours, they will be 272 miles apart. What is Molly's speed?

Let x be Molly's speed. Since Leopold drives 6 miles per hour faster than Molly, his speed is $x + 6$ .

$$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & & & time & & $\cdot$ & & speed & & $=$ & & distance & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & Leopold & & 4 & & $\cdot$ & & $x + 6$ & & $=$ & & $4(x + 6)$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & Molly & & 4 & & $\cdot$ & & x & & $=$ & & $4 x$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & & & & & & & & & & & 272 & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} }} $$

The last column says

$$4(x + 6) + 4 x = 272.$$

Solve for x:

$$\eqalign{ 4(x + 6) + 4 x & = 272 \cr 4 x + 24 + 4 x & = 272 \cr 8 x + 24 & = 272 \cr 8 x & = 248 \cr x & = 31 \cr}$$

Molly's speed is 31 miles per hour.

32. Phoebe invests $2000 in two accounts. One pays 6% simple interest, while the other pays 8% simple interest. At the end of a year, she has earned $128 in interest. How much was invested in each account?

Let x be the amount invested at 6% and let y be the amount invested at 8%. Since there was $2000 invested, I must have $x + y = 2000$ , or $y = 2000 - x$ .

$$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & & & amount invested & & interest rate & & interest earned & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & 6\% account & & x & & 0.06 & & $0.06 x$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & 8\% account & & $2000 - x$ & & 0.08 & & $0.08(2000 - x)$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & total & & & & & & 128 & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} }} $$

The last column says $0.06 x + 0.08(2000 - x) = 128$ . Solve this equation for x:

$$\eqalign{0.06 x + 0.08(2000 - x) & = 128 \cr 100\cdot 0.06 x + 100\cdot 0.08(2000 - x) & = 100\cdot 128 \cr 6 x + 8(2000 - x) & = 12800 \cr 6 x + 16000 - 8 x & = 12800 \cr -2 x + 16000 & = 12800 \cr -2 x & = -3200 \cr x & = 1600 \cr}$$

Then $y = 2000 - 1600 = 400$ . Thus, $1600 was invested at 6% and $400 was invested at 8%.

33. After one interest period, the interest on a $700 investment is $3 greater than the interest on a $500 investment. The $500 is invested at a rate $0.6%$ higher than the rate for the $700 investment. Find the interest rate for each investment.

Let x be the interest rate for the $700 investment. Since the $500 is invested at a rate $0.6%$ higher, the interest rate for the $500 investment is $x + 0.006$ .

Let y be the interest earned by the $500 investment. Since the $700 investment earned $3 more, the $700 investment earned $y + 3$ dollars in interest.

$$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & & & Amount invested & & $\cdot$ & & Interest rate & & $=$ & & Interest earned & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & \$700 & & 700 & & $\cdot$ & & x & & $=$ & & $y + 3$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & \$500 investment & & 500 & & $\cdot$ & & $x + 0.006$ & & $=$ & & y & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} }} $$

The rows give the equations

$$700 x = y + 3 \quad\hbox{and}\quad 500(x + 0.006) = y.$$

Plug $y = 500(x + 0.006)$ into $700 x = y + 3$ and solve for x:

$$\eqalign{ 700 x & = 500(x + 0.006) + 3 \cr 700 x & = 500 x + 3 + 3 \cr 200 x & = 6 \cr x & = 0.03 \cr}$$

The $700 was invested at $0.03 = 3%$ and the $500 was invested at $0.03 + 0.006 = 3.6%$ .

34. The sum of two numbers is 153. The second number is 5 more than 3 times the first number. Find the numbers.

Let x be the first number and let y be the second number. The sum is 153:

$$x + y = 153.$$

The second number is 5 more than 3 times the first number:

$$y = 5 + 3 x.$$

Plug $y = 5 + 3 x$ into $x + y = 153$ and solve for x:

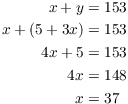
$$\eqalign{ x + y & = 153 \cr x + (5 + 3 x) & = 153 \cr 4 x + 5 & = 153 \cr 4 x & = 148 \cr x & = 37 \cr}$$

The first number is 37 and the second number is $5 + 3 \cdot 37 = 116$ .

35. Calvin Butterball has $4.00 in dimes and nickels. The number of dimes is 5 less than twice the number of nickels. Find the number of dimes and the number of nickels.

Let d be the number of dimes and let n be the number of nickels.

The number of dimes is 5 less than twice the number of nickels, so $d = 2n - 5$ .

There are d dimes and each dime is worth 10 cents, so the dimes are worth $10d$ cents. There are n nickels and each nickel is worth 5 cents, so the nickels are worth $5n$ cents.

Calvin has $4.00 (or 400 cents) all together, so $400 = 10d + 5n$ .

$$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & & & number of coins & & value per coin & & total value & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & dimes & & $d = 2n - 5$ & & 10 & & $10d$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & nickels & & n & & 5 & & $5n$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & all together & & - & & - & & 400 & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} }} $$

Plugging $d = 2n - 5$ into $400 = 10d + 5n$ gives

$$400 = 10(2n - 5) + 5n, \quad 400 = 20n - 50 + 5n, \quad 400 = 25n - 50.$$

Solve for n:

$$\matrix{ & 400 & = & 25n & - & 50 \cr + & 50 & & & & 50 \cr \noalign{\vskip2pt\hrule\vskip2pt} & 450 & = & 25n & & \cr \div & 25 & & 25 & & \cr \noalign{\vskip2pt\hrule\vskip2pt} & 18 & = & n & & \cr}$$

Plugging this into $d = 2n - 5$ gives $d = 36 - 5 = 31$ .

Calvin has 31 dimes and 18 nickels.

36. Sarevok mixes an alloy containing $18%$ silver with an alloy containing $30%$ silver to make 50 pounds of an alloy with $26.4%$ silver. How many pounds of each kind of alloy did he use?

$$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & & & Pounds of alloy & & $\cdot$ & & Percent silver & & $=$ & & Pounds of silver & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & $18\%$ silver & & x & & $\cdot$ & & 0.18 & & $=$ & & $0.18 x$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & $30\%$ silver & & y & & $\cdot$ & & 0.3 & & $=$ & & $0.3 y$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & Total & & 50 & & $\cdot$ & & 0.264 & & $=$ & & 13.2 & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} }} $$

The first and third columns give

$$x + y = 50 \quad\hbox{and}\quad 0.18 x + 0.3 y = 13.2.$$

Multiply the first equation by 0.3 and subtract the second equation:

$$\eqalign{ 0.3 x + 0.3 y & = 15 \cr 0.18 x + 0.3 y & = 13.2 \cr \noalign{\vskip2pt \hrule \vskip2pt} 0.12 x & = 1.8 \cr x & = 15 \cr}$$

Hence, $y = 50 - 15 = 35$ . He used 15 pounds of the $18%$ alloy and 35 pounds of the $30%$ alloy.

37. Silas Hogwinder has some 13-cent stamps, some 17-cent stamps, and some 40-cent stamps. The number of 13-cent stamps is 2 less than the number of 17-cent stamps. The number of 40-cent stamps is 1 more than twice the number of 17-cent stamps. The total value of the stamps is $13.34. Find the number of each type of stamp that Silas has.

Let n be the number of 17-cent stamps.

The number of 13-cent stamps is 2 less than the number of 17-cent stamps, so the number of 13-cent stamps is $n - 2$ .

The number of 40-cent stamps is 1 more than twice the number of 17-cent stamps, so the number of 40-cent stamps is $2n + 1$ .

I put the information into a table:

$$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & & & number of stamps & & $\cdot$ & & cents per stamp & & $=$ & & total value (cents) & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & 13-cent stamps & & $n - 2$ & & $\cdot$ & & 13 & & $=$ & & $13(n - 2)$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & 17-cent stamps & & n & & $\cdot$ & & 17 & & $=$ & & $17n$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & 40-cent stamps & & $2n + 1$ & & $\cdot$ & & 40 & & $=$ & & $40(2n + 1)$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & total & & & & & & & & & & 1334 & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} }} $$

The last column says

$$13(n - 2) + 17n + 40(2n + 1) = 1334.$$

Solve for n:

$$\eqalign{ 13(n - 2) + 17n + 40(2n + 1) & = 1334 \cr 13n - 26 + 17n + 80n + 40 & = 1334 \cr 110n + 14 & = 1334 \cr 110n & = 1320 \cr n & = \dfrac{1320}{110} = 12 \cr}$$

The number of 17-cent stamps is 12, the number of 13-cent stamps is $n - 2 = 12 - 2 = 10$ , and the number of 40-cent stamps is $2n + 1 = 2(12) + 1 = 25$ .

38. How many gallons of a $40%$ alcohol solution and a $30%$ alcohol solution must be mixed together to make 50 gallons of a $32%$ alcohol solution?

Let x be the number of gallons of the $40%$ solution and let y be the number of gallons of the $30%$ solution.

There are 50 gallons all together, so $x + y = 50$ .

x gallons of $40%$ solution contain $40%$ of x, or $0.4 x$ gallons of alcohol.

y gallons of $30%$ solution contain $30%$ of y, or $0.3y$ gallons of alcohol.

The mixture is 50 gallons of a $32%$ solution; it contains $32%$ of 50, or $0.32\cdot 50 = 16$ gallons of alcohol.

Therefore, $0.4 x + 0.3y = 16$ .

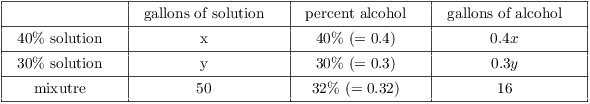
$$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & & & gallons of solution & & percent alcohol & & gallons of alcohol & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & $40\%$ solution & & x & & $40\%$ ($= 0.4$) & & $0.4 x$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & $30\%$ solution & & y & & $30\%$ ($= 0.3$) & & $0.3y$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & mixutre & & 50 & & $32\%$ ($= 0.32$) & & 16 & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} }} $$

Solve $x + y = 50$ to get $y = 50 - x$ . Plug this into $0.4 x + 0.3y = 16$ :

$$0.4 x + 0.3(50 - x) = 16, \quad 0.4 x + 15 - 0.3 x = 16, \quad 0.1 x + 15 = 16.$$

Solve for x:

$$\matrix{ & 0.1 x & + & 15 & = & 16 \cr - & & & 15 & & 15 \cr \noalign{\vskip2pt\hrule\vskip2pt} & 0.1 x & & & = & 1 \cr \div & 0.1 & & & & 0.1 \cr \noalign{\vskip2pt\hrule\vskip2pt} & x & & & = & 10 \cr}$$

Plug this into $y = 50 - x$ to get $y = 50 - 10 = 40$ .

You need 10 gallons of the $40%$ solution and 40 gallons of the $30%$ solution.

39. How many pounds of dried fruit worth $7 per pound must be mixed with 4 pounds of peppermint ketchup worth $3.50 per pound to make a mixture worth $5 per pound?

Let x be the number of pounds of dried fruit needed. Fill in the table:

$$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & & & pounds & & price per pound & & total price & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & dried fruit & & x & & 7 & & $7 x$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & peppermint ketchup & & 4 & & 3.5 & & 14 & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & \cr & mixture & & $x + 4$ & & 5 & & $7 x + 14$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} }} $$

The last line says:

$$5(x + 4) = 7 x + 14.$$

Solve for x:

$$5(x + 4) = 7 x + 14, \quad 5 x + 20 = 7 x + 14, \quad 6 = 2 x, \quad x = 3.$$

Therefore, 3 pounds of dried fruit are required.

40. Bonzo divides $1000 up between two accounts. The first account pays $6%$ annual interest, while the second pays $8%$ annual interest. After one year, the interest earned by the $8%$ account was $31 more than the interest earned by the $6%$ account. Find the amounts that were invested in the two accounts.

Suppose x dollars were invested in the $6%$ account and y dollars were invested in the $8%$ account.

$$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & & & amount invested & & $\cdot$ & & interest rate & & $=$ & & interest earned & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & $6\%$ account & & x & & $\cdot$ & & 0.06 & & $=$ & & $0.06 x$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & $8\%$ account & & y & & $\cdot$ & & 0.08 & & $=$ & & $0.08y$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & total & & 1000 & & & & & & & & & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} }} $$

There was a total of $1000 invested:

$$x + y = 1000.$$

The interest earned by the $8%$ account was $31 more than the interest earned by the $6%$ account:

$$0.08y = 31 + 0.06 x.$$

Solve the first equation for y;

$$\eqalign{ x + y & = 1000 \cr x + y - x & = 1000 - x \cr y & = 1000 - x \cr}$$

Plug this into $0.08y = 31 + 0.06 x$ , clear the decimals, then solve for x:

$$\eqalign{ 0.08y & = 31 + 0.06 x \cr 0.08(1000 - x) & = 31 + 0.06 x \cr 100 \cdot 0.08(1000 - x) & = 100 \cdot 31 + 100 \cdot 0.06 x \cr 8(1000 - x) & = 3100 + 6 x \cr 8000 - 8 x & = 3100 + 6 x \cr 8000 - 8 x + 8 x - 3100 & = 3100 + 6 x + 8 x - 3100 \cr 4900 & = 14 x \cr 4900/14 & = 14 x/14 \cr 350 & = x \cr}$$

Therefore, $y = 1000 - 350 = 650$ . Thus, $350 was invested in the $6%$ account and $650 was invested in the $8%$ account.

41. Gordon Freeman goes on a car trip of 345 miles that takes a total of 6 hours. He averages 54 miles per hour for the first part of the trip; after a rest stop, he continue his drive and averages 60 miles per hour for the second part of the trip. How long were the two parts of the trip?

Let x be the time for the first part of the trip and let y be the length of the second part of the trip.

$$\vbox{\offinterlineskip \halign{& \vrule # & \strut \hfil \quad # \quad \hfil \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & & & Time & & $\cdot$ & & Speed & & $=$ & & Distance & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & First part & & x & & $\cdot$ & & 54 & & $=$ & & $54 x$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & Second part & & y & & $\cdot$ & & 60 & & $=$ & & $60 y$ & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr & Whole trip & & 6 & & & & & & & & 345 & \cr height2pt & \omit & & \omit & & \omit & & \omit & & \omit & & \omit & \cr \noalign{\hrule} }} $$

I get the equations

$$x + y = 6 \quad\hbox{and}\quad 54 x + 60 y = 345.$$

Multiply the first equation by 60, subtract the second, and solve for x:

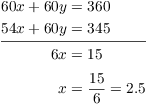
$$\eqalign{ 60 x + 60 y & = 360 \cr 54 x + 60 y & = 345 \cr \noalign{\vskip2pt \hrule \vskip2pt} 6 x & = 15 \cr \noalign{\vskip2pt} x & = \dfrac{15}{6} = 2.5 \cr}$$

Then $x + y = 6$ gives $2.5 + y = 6$ , so $y = 3.5$ . The first part took 2.5 hours and the second part took 3.5 hours.

Growth is barely controlled damage. - James Richardson

Contact information

Bruce Ikenaga's Home Page

Copyright 2020 by Bruce Ikenaga